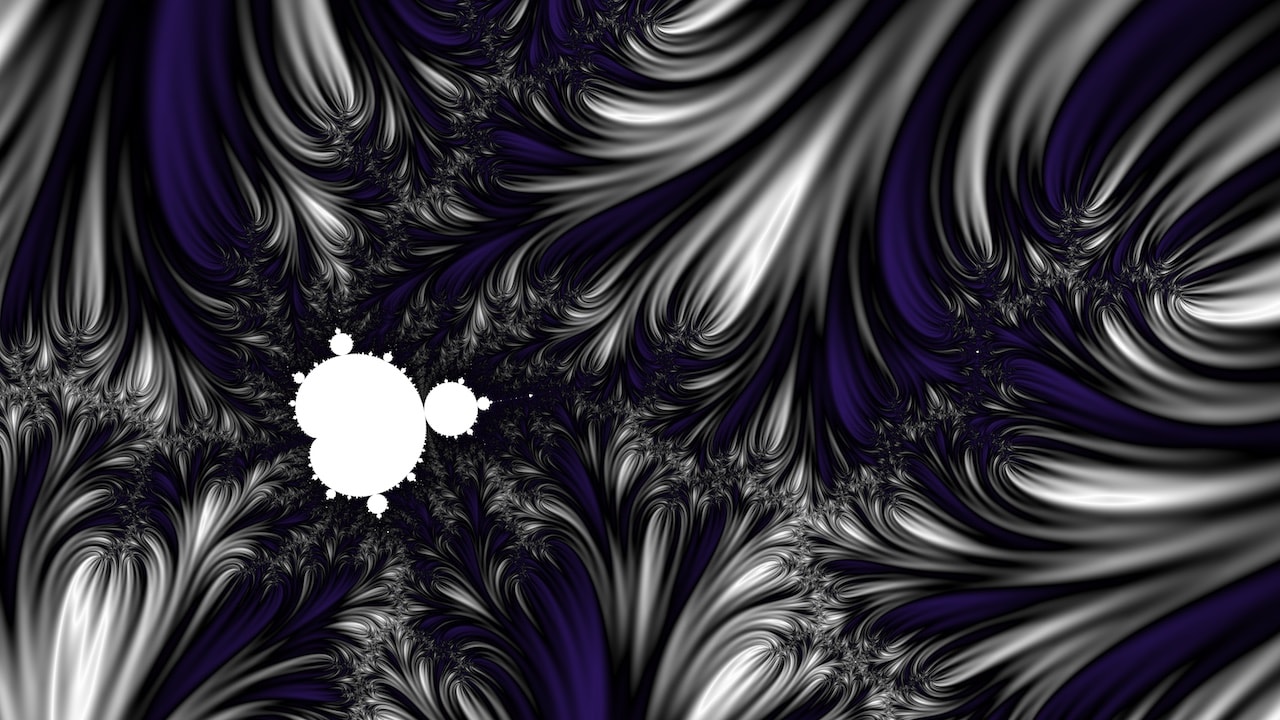
March 15, 2023: "Arrival" by me

The well-known Stripe Average Coloring is now available for use in Very Plotter's Mandelbrot set viewer! This is an "easy" location that shows some of what Stripe Average Coloring can do.

Click here to view this location in Very Plotter.

Available renders:

• Arrival-3200x1800.png

Location:
Re: -0.0349877428384
Im: -0.984380520487
Magnification: 1.33333333333e2 (where 1.0 fits entire Mandelbrot set into the window)
Scale: 3.99999999999e4 (pixels/unit, for renders seen here)
Iterations: 50000

March 16, 2023: "Arctic" by me

This is another simple, shallow location, but it uses the well-known (but new to Very Plotter) Stripe Average Coloring mode to reveal lots of detail in what would look like "empty" areas with normal iteration coloring.

Click here to view this location in Very Plotter.

Available renders:

• Arctic-3200x1800.png
• Arctic-2560x1440.png

Location:
Re: -0.160185973664
Im: 1.03805097785
Magnification: 4.84851001083e3 (where 1.0 fits entire Mandelbrot set into the window)
Scale: 1.45455300324e6 (pixels/unit, for renders seen here)
Iterations: 1000

April 17, 2023: "Darkstar" by me

Click here to view this location in Very Plotter.

Available renders:

• Darkstar-3200x1800.png

Location:
Re: -0.12702288604303376058504939571344674642157173
Im: -0.98728474138900681012772183234313984426625069
Magnification: 1.5e24 (where 1.0 fits entire Mandelbrot set into the window)
Scale: 1.8e27 (pixels/unit, for renders seen here)
Iterations: 30000

April 18, 2023: "Infinite-Depths" by me

This is a composite of an iteration coloring image and a separate stripe average coloring image that used a grayscale gradient. Using Photoshop, I set the stripe layer to 15% opacity with the "Pin Light" blending mode. I also made a slight levels and contrast adjustment to the composite image.

Click here to view this location in Very Plotter.

Available renders:

• Infinite-Depths-3200x1800.png

Location:
Re: -0.743643927058028316631291361415826008739702757197807860510631347994998874371449081
Im: 0.131825980877906947146424011940025164151924022214318464431311547636916892789256531
Magnification: 1.99903333333e52 (where 1.0 fits entire Mandelbrot set into the window)
Scale: 2.39883999999e55 (pixels/unit, for renders seen here)
Iterations: 120000

May 4, 2023: "Untitled 8" by Me

This is another composite image, this time of a relatively shallow location. The stripe average coloring layer is blended on top of the base iteration-colored layer. The stripe average coloring layer (which can be viewed here) is at 65% opacity using the "Pin Light" blending mode.

Click here to view this location in Very Plotter.

Available renders:

• Untitled-8-3200x1800.png

Location:
Re: -0.843233764530751513324611729773572725750160827
Im: 0.22381804155806995913989829482160200044199335
Magnification: 1.46484375e25 (where 1.0 fits entire Mandelbrot set into the window)
Scale: 1.7578125e28 (pixels/unit, for renders seen here)
Iterations: 45000

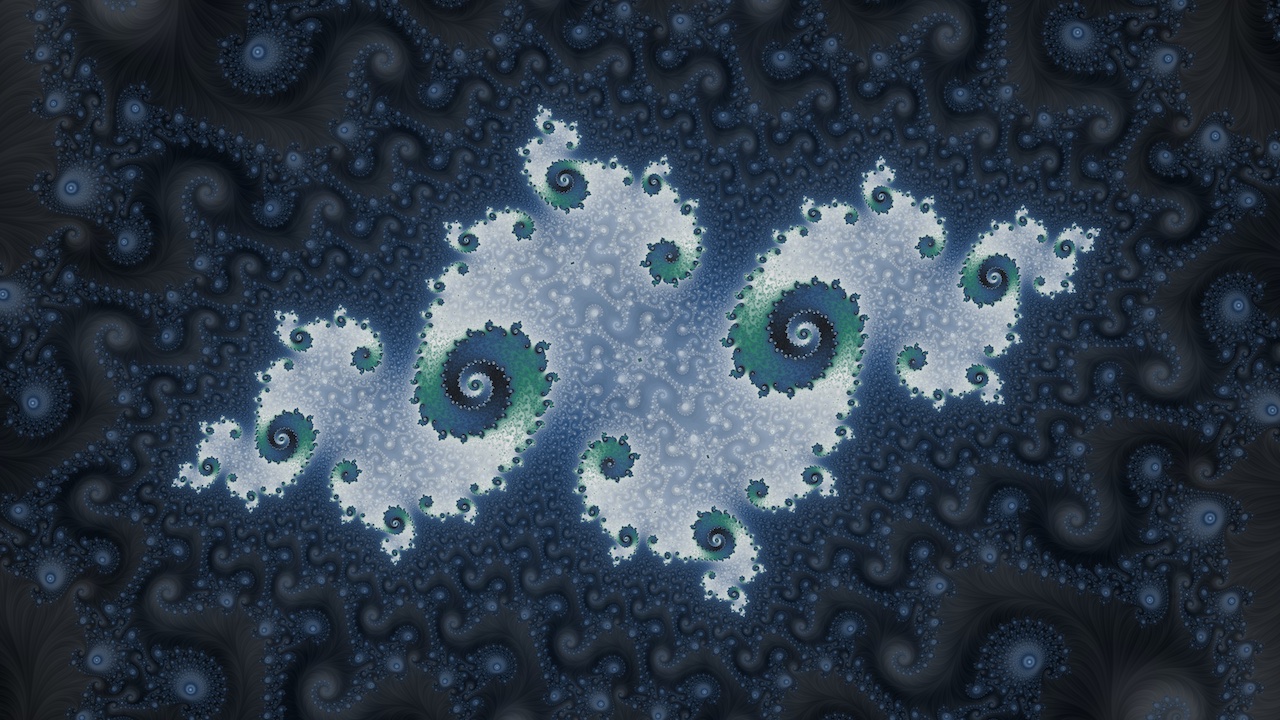
May 9, 2023: "Mandelbrot Deep Julia Morphing 31" by Microfractal

This is a location discovered by "Microfractal" and published on Deviant Art. I'm not sure why my image is a mirror of the Deviant Art version, but perhaps the imaginary component of the location is missing a minus sign.

I used this very deep location for testing BLA (Bivariate Linear Approximation) rendering. I think this is the deepest location I've ever rendered using Very Plotter. It took several days to render with BLA, and I think it would have taken at least twice as long using Series Approximation. Unlike the other renders in this gallery, this was not done at a high resolution (or else it would have taken longer to render!) so it looks a bit rough and grainy. Perhaps someday Very Plotter (or computer hardware) will improve to the point where this location can be rendered quickly. In the meantime, I'd recommend other software (like Kalles Fraktaler) for viewing this location.

Click here to view this location in Very Plotter.

Available renders:

• Mandelbrot-Deep-Julia-Morphing-31-1600x900.png

Location:
Re: -1.94154386426666073385494419139602817911002190783983806486255995390970564901841266654110342825040027833437400890080285153552529327388232371146283717027668881590861362991408508818382192753637902940013651676928079154959840543639128710705789946261966322401536496779684342233569702585514036404569468368027709623003705407121586854803770468577214029441021980626839467735283281182663319948425162501139757726233019859661104651575768057772089890530536093546336903563326842789114624929967044053119714067132315872304777456576801951933320843131435868568417931243209070672982581424895069161511755346174216235385885774648591314732196413689311503556588612727187269408874241691974841087855881441722561548154608791302804221857246655758140119158227702095944162158523180675710335847354760536529095575520493785351579199840120211532761876756343130808435230665830020934938543221112081079909739139542994199217699113323641765080079007385893739678573743535741773511560401049413603608150466977026779033151892911965595117342808620118692133269782138546471855839497910778747195417038495889140353552407452462813979865884247117219964609626521955405883942216120822382390240301518825634451113002797903958517780042374768669868605876961997371941004054340838582253877498107382335786449824606444004049030482264919457882439320013661467456591605894357554801802587618779544348760296765593619667730003550914436687920459780348984199661509102043839301448365028378964509455099138460773025273107651621339672997109207592844405085921355044477761954888096227770110133580290394997631024115310189617576320280301943133838300075519310361941099131596321800889020233797326469236132725205057764663720710328551666848930753609443359331448130481041900710459691431714913426703252751947571967544670147394307762368018437507996150024757785995051757400629127935386
Im: 0.0000892293339542148802272919829589858035157573184985491365534013497345807545516142511469397149227340254773284727735761562371422707868600251597663489406963042316241464024727277383914467526119307103666479936023211178563973072348476624466716957624802981233723506239398422794680891860496058103906488118267738131742630907369904328623619542954811212850982953513536315164703408712552692095279609420680275306429101648926406275381107379180907707352263859347328016787764472509420142628506266103582236137609984767003142310239389456832848062120259156957805594956209430304776643728042483525019600600524987444945171539843757282351045893033312998396154950430039383326640463532644580094068611392337878766755748669395133717213659853675344530279894193194970966181411023333634050346491682169078160619901053509115040890613086868771310251761807249318903963806396677209237220915874371487343505175925749276986089526041420475965999730124291818837015269557404911672743115914752807530580744001871407992010328708110165611600502841642819890286618657551516238241984988123010201511719788299877887464701980936133340884217631999170602503865525907133147554401909151134753881604517170419895018205554070493063143570519191651341914131478022040900027393959614371774452159183649357373611813933649181034898584048409764177590626963963739334568374899911953794270807407737420757720424281568070343108466249711669614733043869539689948379942106459090499557342715575511429200094759829317273504456001382020853064149767176560041825645958181314452312930161303545310845428551923614318965269773077821275030276642958427531272828515379072486562852557909567639681274345070907241110253800158209455298867022577798925055205722291510540395033930802226561727219875181190640009570845824522532447566320440377845742731390068739472139795727332157605065081990993127837
Magnification: 2.2e1744 (where 1.0 fits entire Mandelbrot set into the window)
Scale: 6.6e1746 (pixels/unit, for renders seen here)
Iterations: 8500000

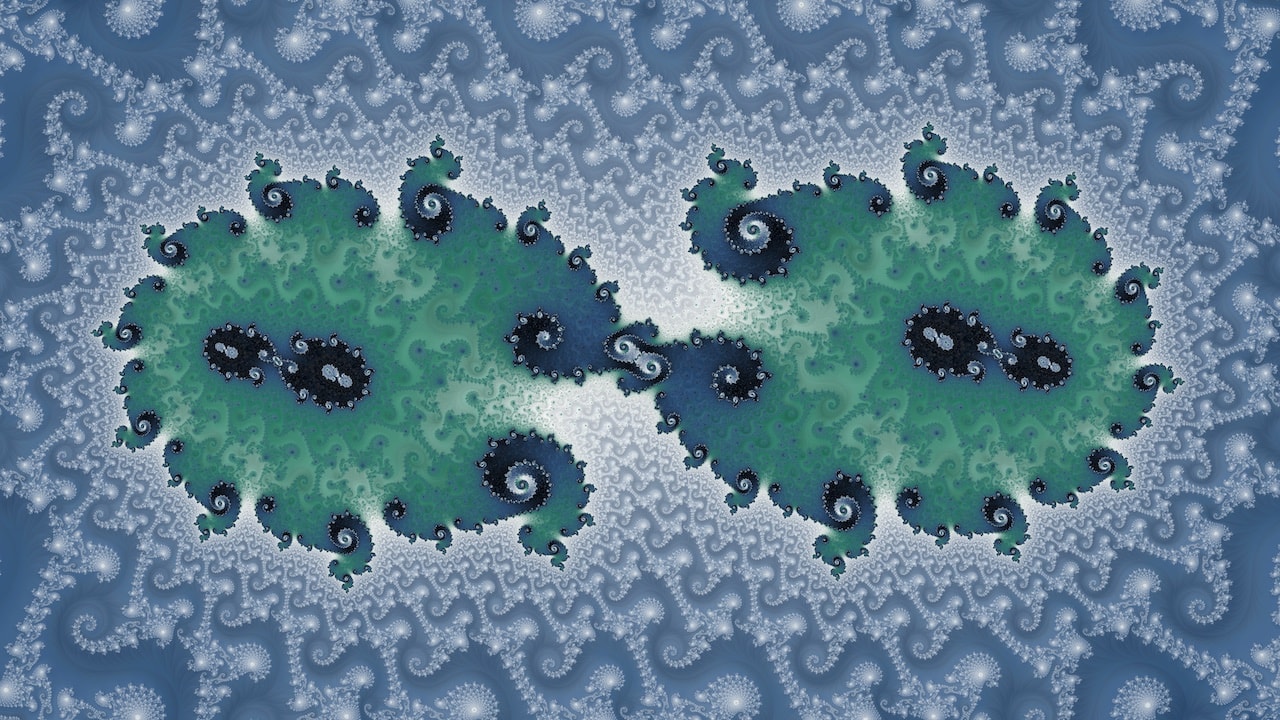
May 15, 2023: "Islands" (2023 version) by Wolfgang Beyer

This is the same location as the first image in my Mandelbrot set gallery, from 2021. As I said in the description for that render, this is the image that kicked off my Mandelbrot set journey. It appears on the Mandelbrot set Wikipedia article (details of this particular image are here).

For this render, I created a 4-panel image that I stiched together in Photoshop. Each image is a composite of a stripe coloring image (at 22% opacity with the "Luminosity" blend mode) on top of the iteration color image. The stripe coloring can be viewed here. I went overboard and ended up with a 1.1 gigapixel render at 45,091 x 25,364 resolution. Needless to say it's too large to share here as a download, but I'll think about how to share it.

Among the renders listed below I have a still very large 11,273 x 6,341 render, the usual 3,200 x 1,800 render, and a 2x 6,400 x 3,600. I've also included "no stripe" renders at 3,200 x 1,800 and also 6,400 x 3,600.

Click here to view this location in Very Plotter.

Available renders:

• Wolfgang-Beyer-Islands-2023-3200x1800.png
• Wolfgang-Beyer-Islands-2023-6400x3600.png
• Wolfgang-Beyer-Islands-2023-11273x6341.png
• Wolfgang-Beyer-Islands-2023-no-stripe-3200x1800.png
• Wolfgang-Beyer-Islands-2023-no-stripe-6400x3600.png

Location:
Re: -0.743643927057876963
Im: 0.13182598087768664078
Magnification: 3.16666666666e10 (where 1.0 fits entire Mandelbrot set into the window)
Scale: 9.5e12 (pixels/unit, for renders seen here)
Iterations: 20000

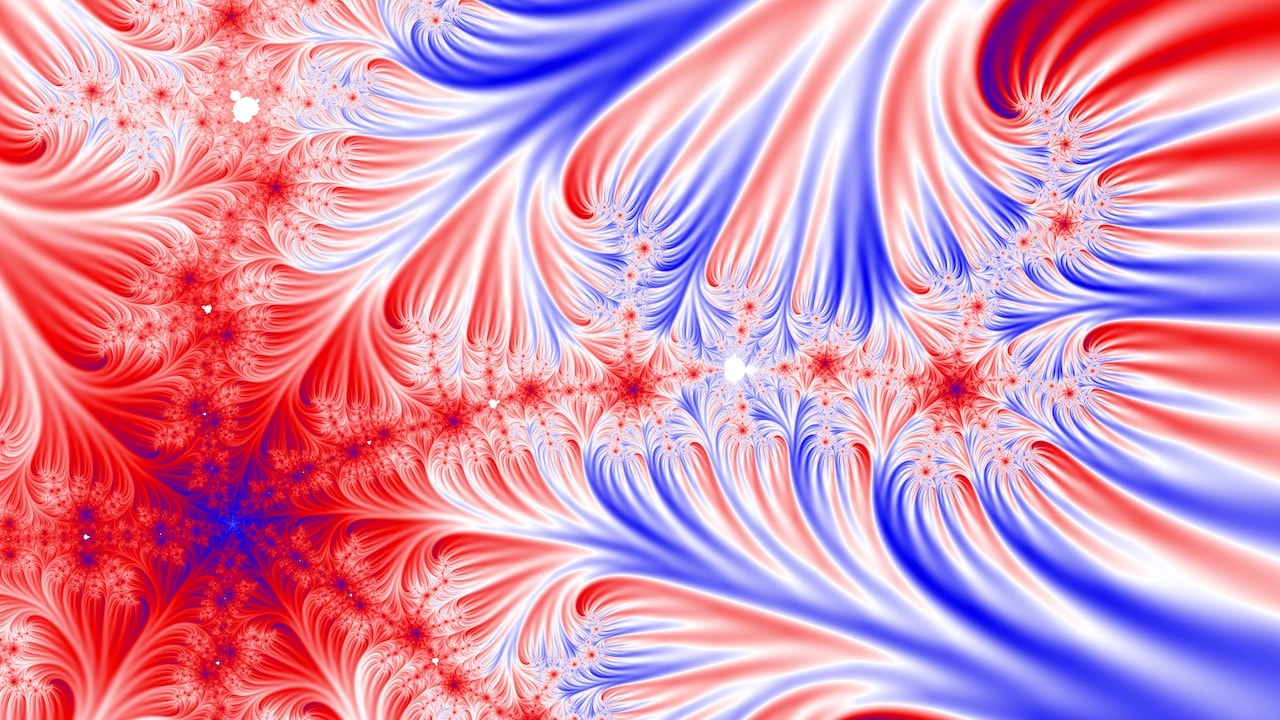
July 4, 2023: "Stars and Stripes 2023" by me

In this image, we have 5-sided "stars" and bold red, white, and blue "stripes" created with Stripe Average Coloring. Happy 4th of July!

Click here to view this location in Very Plotter.

Available renders:

• Stars-and-Stripes-2023-3200x1800.png

Location:
Re: 0.453356524534
Im: 0.348110803291
Magnification: 1.1e2 (where 1.0 fits entire Mandelbrot set into the window)
Scale: 1.32e5 (pixels/unit, for renders seen here)
Iterations: 1776

October 31, 2023: "Halloween 2023" by me

I saw this spider web while working on Stripe Average Coloring earlier this year. Halloween colors and orange minibrots add to the fun. To get orange minibrots, open your browser's JavaScript console and enter "bgColorSchemes['w'] = 'rgba(166,74,19,0)';" and cycle the minibrot/background color with the "b" key.

Click here to view this location in Very Plotter.

Available renders:

• Halloween-2023-3200x1800.png

Location:
Re: -1.9408510363187962822
Im: -0.00067213130677203846807
Magnification: 1.9e6 (where 1.0 fits entire Mandelbrot set into the window)
Scale: 4.56e9 (pixels/unit, for renders seen here)
Iterations: 25,000